No. 600 - A regular polyomino , an hexomino (with all 35 pieces that can be constructed of 6 squares) in a rectangular tray with a very small protuberance. It's very peculiar these pieces won't fit in any rectangular surface (like 14 x 15 = 210). Golomb proved this in a simple and understandable way in his book.
Only one puzzle of the serie is more difficult than this one. Still Tenyo claims the existance of over 1.000.000.000 (!) different solutions! I believe they stopped the computer at this point, the real number must be a factor higher. Probably by far the highest number of solutions in de Beat The Computer series!




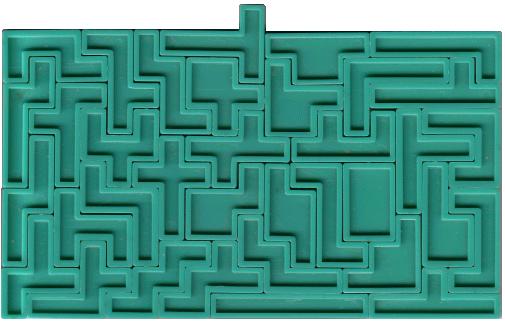
With the next plan I found over 10.000.000 solutions myself.
The rectangles at the left can be rotated independantly and be swapped for each other as well.



With this invention Gerard Traarbach found over 1.000.000.000 (!) computer solutions by hand.
The squares at the bottom can be rotated independantly and be swapped.



Download
You can download my Hexomino solver (written in Delphi 6 for Windows) here.
The solver can show all solutions of any puzzle shape.
Free Hexomino solver

more 'Beat The Computer' puzzles

More about polyominos

Play this puzzle yourself