When you are working on the Square Puzzle you can often meet the sequence of Fibonacci. This is the number serie 1 2 3 5 8 13 21 34 ... Every next number is the sum of the two preceding numbers.
With the shell or spiral of Fibonacci you can tile rectangles with squares leaving very few open space!


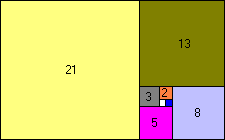
The larger this spriral, the more it nears the 'golden mean'.

You can also meet the 'wrongly started' sequence of Fibonacci, if you omit square 2.


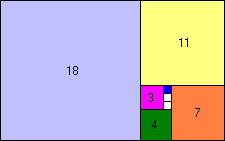
Also this one nears the 'golden mean'.

When you already have a perfect rectangle or square, with Fibonacci you're able to create an infinite number of perfect rectangles (never a square)


Ron Knott's complete site about Fibonacci
Willem Pijnappels Fibonacci pages

back to the square puzzle